31 Jul 2013
Irish MO 1988 P1 Q9: Lower Secondary Mathematics Competition Question
The year 1978 was “peculiar" in that the sum of the numbers formed with the first two digits and the last two digits is equal to the number formed with the middle two digits, i.e., 19 + 78 = 97.
What was the last previous "peculiar" year, and when will the next one occur?
[ Irish MO 1988 Paper 1 Question 9]
IrMO 2003 P1 Q1: Middle Secondary Mathematics Competition Question
Find all integer solutions of the equation
(m2 + n)(m + n2) = (m + n)3
[From IrMO 2003, Paper 1, Question 1]
(m2 + n)(m + n2) = (m + n)3
[From IrMO 2003, Paper 1, Question 1]
30 Jul 2013
Smallest Quadrilateral: Lower Secondary Mathematics Competition Question
Triangles ABC and BCD share the side BC. Both are right-angled with distinct integer sides (apart from, obviously, BC). What is the smallest area of the non-degenerate quadrilateral ABDC?
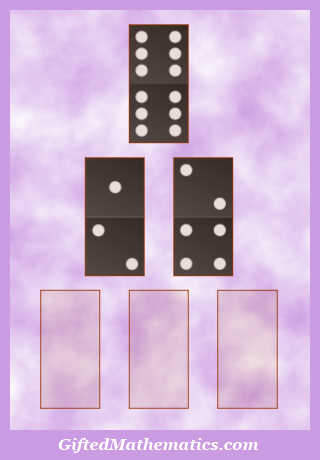
Domino Triangles: Winner of PMQ20: Middle Secondary Mathematics
Before launching into the question itself, note that this question was the winner of PMQ20, where you were asked to design your own question based around dominoes. A big “Thank You” to Chris Breederveld for submitting the puzzle below.
The Question
Take one full set of dominoes and remove those tiles with blanks, leaving you with 21 distinct tiles. Orient each tile vertically so that it denotes a rational number. For example, the tile [5/2] can be used as the fraction 5/2 or as 2/5. The aim is to arrange six tiles into a triangular grid in such a way that a tile-fraction is the sum of the two tile-fractions immediately below it.
Given that the tile at the top of the triangle is [6/6], how many solutions can you find? Treat reflections as one unique solution.
The diagram below shows the start of one possible solution. Note that the only restriction is that the [6/6] must be at the top.
26 Jul 2013
Prize Maths Quiz: Nine Rooms Puzzle (PMQ29)
You are in a network of rooms laid out in a 3 by 3 grid (as shown in the diagram). Each room has a door connecting it to rooms adjacent to it. There are 12 doors in total and all are open at the start of the game. You are placed in one of the corner rooms and your aim is to visit each room once. When you leave a room, all the doors connected to that room are automatically closed and locked. You may finish your route at any convenient room.
In the middle of each room, there is a gold coin placed on a table. You must enter a room in order to pick up each coin. If you have successfully collected all nine gold coins, you will be magically teleported to freedom!
The diagram shows one possible winning route. Starting from the same room in the top left corner of the network, how many different routes are possible so as to visit each room once?
Now, that was far too easy! The real game is slightly more challenging. In the first room, next to the gold coin, are two dodecahedral dice, each numbered 1 to 12, and a small map of the network. Each door in the network has a number on it from 1 to 12 inclusive and the map shows the location of each numbered door. You roll the two dice until you have two distinct numbers; you may only roll again if the two numbers are the same. You will then hear the sound of two doors being locked closed; they are the doors that correspond to the two numbers on the dice.
What is the probability that you can still pick up all nine gold coins, even after the two doors have been locked?
25 Jul 2013
Black-Balled: Upper Secondary Mathematics Competition Question
Imagine you have two identical boxes. Inside each box are four balls; the balls are identical to the touch but seven of them are coloured white and one is black. You randomly select one box then, without looking inside, randomly pick out one ball and put it to one side. Repeat this process until one of the boxes is empty.
What is the probability that the black ball is still inside the other box?
Although physically difficult to actually play this game randomly, you could select which box to open by flipping a coin.
[Adapted from the Math Prize for Girls 2012]
24 Jul 2013
Knucklebones: Middle Secondary Mathematics Competition Question
A) Because of their peculiar shape, the bones only had four numbered faces. Traditionally, these had the values 1, 3, 4 and 6. The approximate probabilities of rolling each number were in the ratios 1 : 3 : 3 : 1. Playing with 3 such knucklebones, and assuming these probabilities are exact, what is the probability of rolling a sum total of 8?
B) From what we know of the ancient Greeks and Romans, they tended to play with 4 knucklebones and particular throws had distinctive names. A Venus (or Aphrodite) was a roll in which all four different numbers appeared, (1, 3, 4, 6); a Dog was the lowly (1, 1, 1, 1)! What is the probability of rolling a Venus?
23 Jul 2013
Find ABCDE: Lower Secondary Mathematics Competition Question
A five-digit number ABCDE is made using one each of the numerals 1, 2, 3, 4 and 5. The three-digit number ABC is divisible by 3, the number BCD is divisible by 4 and CDE is divisible by 5. Find all possible solutions of ABCDE and calculate their sum.
22 Jul 2013
Knucklebones: Upper Primary Mathematics Competition Question
Knucklebones were originally made from part of the ankle of a sheep; they were also known by their more anatomically correct name of astragaloi. They survive to this day in the children’s game known as Jacks. However, knucklebones were also used as dice.
Because of their peculiar shape, knucklebones only had four numbered faces, with the values 1, 3, 4 and 6. Ancient Greeks and Romans seem to have enjoyed playing a game that used four such knucklebones as dice. If we add together the values on the four exposed faces, which numbers between 1 and 24 inclusive can never be rolled?
Because of their peculiar shape, knucklebones only had four numbered faces, with the values 1, 3, 4 and 6. Ancient Greeks and Romans seem to have enjoyed playing a game that used four such knucklebones as dice. If we add together the values on the four exposed faces, which numbers between 1 and 24 inclusive can never be rolled?
19 Jul 2013
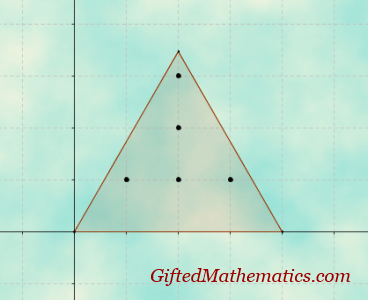
Prize Maths Quiz: Points in a Triangle (PMQ28)
An equilateral triangle lies in the plane with two of its vertices at the points (0, 0) and (n, 0), where n is an integer. Determine the number of points (x, y) with integer coordinates that lie in the interior of the triangle.
Your final answer should be a formula that relates the total number of lattice points (x, y), call it N, to the x-coordinate, n. Note also that the lattice points must lie within the triangle and not along its perimeter.
Have fun!
18 Jul 2013
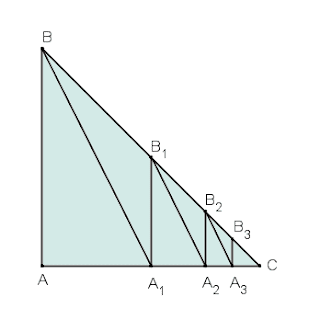
Sum of Diagonals: Upper Secondary Mathematics Competition Question
The figure ABC is a right-angled triangle with lengths AB = AC = 4. Point A1 is the mid-point of the line AC; the point A2 is the mid-point of the line segment A1C, and so on. The line segment A1B1 is perpendicular to AC and intersects the line BC at point B1; similarly A2B2 is perpendicular to AC and intersects BC at B2, and so on.
This construction gives us the diagonal line segments of lengths BA1, B1A2, B2A3 and so on. Calculate the sum of the infinite series
D = BA1 + B1A2 + B2A3 + B3A4 + ...
Hence, if H is the length BC, find the value of D/H.
17 Jul 2013
Decimal Expansions of Reciprocals: Middle Secondary Mathematics Competition Question
The Golden Ratio φ (phi) has the peculiar property that the decimal expansion of φ (1.6180339887...) is identical to that of its reciprocal, 1/ φ (0. 6180339887...).
How many other pairs of positive real numbers below 10 have this property?
Show one of your solutions.
A Diophantine Equation with Factorials: Upper Secondary Mathematics Competition Question
This problem has appeared in a variety of Olympiad-level competitions. There are not many solutions, but you also need to prove that you have all of them.
Find all pairs of positive integers (n, k) such that n! = (n + 1)k - 1.
Find all pairs of positive integers (n, k) such that n! = (n + 1)k - 1.
General Continued Squareroots: Middle Secondary Mathematics Competition Question
This is the general case of the previous question.
Let x and N both be positive integers, such that:
Calculate the sum of the first five smallest distinct solutions of N.
Let x and N both be positive integers, such that:
Calculate the sum of the first five smallest distinct solutions of N.
15 Jul 2013
Order of Powers: Upper Primary Mathematics Competition Question
Place these numbers in decreasing order of size.
8^1, 7^2, 6^3, 5^4, 4^5, 3^6, 2^7, 1^8
Which is the highest number?
8^1, 7^2, 6^3, 5^4, 4^5, 3^6, 2^7, 1^8
Which is the highest number?
13 Jul 2013
Prize Maths Quiz: King Arthur and the Round Table Puzzle (PMQ27)
King Arthur was slumped on his throne, glaring at his squabbling knights. Time for another quest, he thought.
"Right! All of you sit down! Time for one of you to go in search of the fabled Grail."
The knights groaned; it echoed round the chamber, sounding like it had been emitted by a giant bear. As the knights shuffled their way to the Round Table, Sir Rod the Obtuse spoke what was on all their minds.
"Great King, how will you decide which of us gallant knights shall set forth on this onerous quest?"
"I think 'gallant' is exhibiting a rash of pride, Sir Rod. Nevertheless, to answer your question, I shall use the usual method. All I am willing to divulge is that I shall be using... two dice."
The consternation that followed was merely a manifestation of their collective inner turmoil. Mathematical acuity had never been on the list of personal qualities necessary for knighthood. Merlin had the brains, and most knights were more than happy not to tread on Merlin's turf. Amidst the anxious clatter, Sir Rod the Obtuse had a quiet word with Merlin, who was taking an aloof stance to the proceedings. Merlin nodded in agreement and the two men parted.
The Round Table was designed so that the chairs were numbered in sequence 0, 1, 2, 3, ... n, where n was the number of knights present. The chair numbered 0 was always empty, ready for the return of a knight who would finally find the Grail. King Arthur sat on an unnumbered throne.
Once all the knights were seated, Arthur would take out some dice from a box and roll them to get a number, k. Arthur would then count around the Table, starting at 0, and eliminate the knight seated in every k-th chair. He would continue in this fashion until only one chair was left. If the last remaining chair was the 0-chair, then nobody would set out to find the Grail - but King Arthur still enjoyed the sport of making his knights sweat!
For example, if Arthur rolls a 3, and there are only 4 knights present, then the chairs would be numbered (0, 1, 2, 3, 4) and sequence of eliminated chairs would be (2, 0, 4, 1, 3). The knight sitting in chair number 3 would be packing his bags and saddling his horse.
On this particular day, there were 12 knights present and King Arthur chose to roll two standard six-sided dice. Sir Rod the Obtuse had no intention of riding off to his doom, nor of returning to find himself dispossessed. Which numbered chair should he sit in to avoid being chosen for the Grail quest?
"Right! All of you sit down! Time for one of you to go in search of the fabled Grail."
The knights groaned; it echoed round the chamber, sounding like it had been emitted by a giant bear. As the knights shuffled their way to the Round Table, Sir Rod the Obtuse spoke what was on all their minds.
"Great King, how will you decide which of us gallant knights shall set forth on this onerous quest?"
"I think 'gallant' is exhibiting a rash of pride, Sir Rod. Nevertheless, to answer your question, I shall use the usual method. All I am willing to divulge is that I shall be using... two dice."
The consternation that followed was merely a manifestation of their collective inner turmoil. Mathematical acuity had never been on the list of personal qualities necessary for knighthood. Merlin had the brains, and most knights were more than happy not to tread on Merlin's turf. Amidst the anxious clatter, Sir Rod the Obtuse had a quiet word with Merlin, who was taking an aloof stance to the proceedings. Merlin nodded in agreement and the two men parted.
The Round Table was designed so that the chairs were numbered in sequence 0, 1, 2, 3, ... n, where n was the number of knights present. The chair numbered 0 was always empty, ready for the return of a knight who would finally find the Grail. King Arthur sat on an unnumbered throne.
Once all the knights were seated, Arthur would take out some dice from a box and roll them to get a number, k. Arthur would then count around the Table, starting at 0, and eliminate the knight seated in every k-th chair. He would continue in this fashion until only one chair was left. If the last remaining chair was the 0-chair, then nobody would set out to find the Grail - but King Arthur still enjoyed the sport of making his knights sweat!
For example, if Arthur rolls a 3, and there are only 4 knights present, then the chairs would be numbered (0, 1, 2, 3, 4) and sequence of eliminated chairs would be (2, 0, 4, 1, 3). The knight sitting in chair number 3 would be packing his bags and saddling his horse.
On this particular day, there were 12 knights present and King Arthur chose to roll two standard six-sided dice. Sir Rod the Obtuse had no intention of riding off to his doom, nor of returning to find himself dispossessed. Which numbered chair should he sit in to avoid being chosen for the Grail quest?
11 Jul 2013
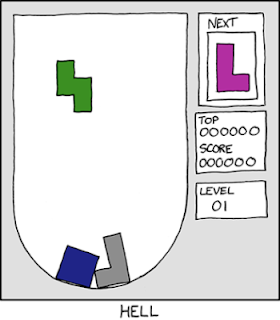
Tetris Hell: Middle Secondary Mathematics
In a week of pentominoes, there is a little game that uses tetrominoes – Tetris. Now, as we have seen, there are 12 free pentominoes. As each pentomino has an area of 5 unit squares, all 12 pentominoes have a combined area of 60 square units. The problem of tiling a rectangle of 60 square units with one each of the 12 pentominoes has numerous solutions.
In contrast, there are just 5 free tetrominoes, each obviously has an area of 4 square units. However, it is impossible to tile any rectangle of 20 square units using one each of all 5 tetrominoes.
Why is this impossible?
ITYM 2013 Pentomino Problem: Upper Secondary Mathematics Competition Question
This is the final part of our Pentomino Week. It is said that every pentomino can tile the plane, meaning that we can do so with no overlaps or gaps – as a counter-example, a regular pentagon cannot tile the plane without gaps. However, because the plane is considered infinite, such a tiling does not take into account whether the boundary of the tiling is rectangular or ‘crinkly’. A harder problem is to establish the conditions whereby the pentominoes can tile various rectangles.
The Question
1. Consider the L pentominoes, that is L and L’ together with their rotations.
a) For which n ϵ N is it possible to tile a 5 × n rectangle (to cover it without overlaps and without gaps)?
b) Find all m, n ϵ N for which one can tile an m × n rectangle.
c) In general, given positive integers m and n, what is the maximal number of L pentominoes that can be placed into an m × n rectangle along grid lines and without overlap?
2. Try the same problems for other types of pentominoes. If a pentomino has a chiral partner, then treat them together
The above question has been adapted from the International Tournament of Young Mathematicians 2013. The simpler parts of the original question have been covered here, here and here. It is not a quick question and, remember, that the ITYM questions may well have no known general solutions! How far you can get into it is the important thing.
The Question
1. Consider the L pentominoes, that is L and L’ together with their rotations.
a) For which n ϵ N is it possible to tile a 5 × n rectangle (to cover it without overlaps and without gaps)?
b) Find all m, n ϵ N for which one can tile an m × n rectangle.
c) In general, given positive integers m and n, what is the maximal number of L pentominoes that can be placed into an m × n rectangle along grid lines and without overlap?
2. Try the same problems for other types of pentominoes. If a pentomino has a chiral partner, then treat them together
The above question has been adapted from the International Tournament of Young Mathematicians 2013. The simpler parts of the original question have been covered here, here and here. It is not a quick question and, remember, that the ITYM questions may well have no known general solutions! How far you can get into it is the important thing.
Pentominoes in a Square: Middle Secondary Mathematics Competitions Question
It is easy to look up that there are 12 free pentominoes. The standard labelling is supposed to approximate to the shape of the tiles and is given in the diagram below. The term ‘free’ means that each tile can be both rotated and reflected. However, if the situation does not allow for reflections – such as Tetris clones – then tiles F, L, N, P, Y and Z have mirror-images that are not identical with themselves by any rotation, yielding 6 new tiles F’, L’, N’, P’, Y’ and Z’. We thus end up with 18 one-sided, or flat, pentominoes. We have already seen in our previous questions that some of these tiles have their own letters (such as P and Q), but I think it is less confusing to just label them as the chiral partners of the originals.
The Question
Take the 18 flat pentominoes and remove the tiles I, L, L’, P and P’; those that make very simple rectangles. Now, tile a 5x5 square with 5 different pentominoes so that there are no gaps or overlaps. How many solutions are there?
Solutions with rotational symmetry count as just one distinct layout, but reflections count as two solutions if they use different flat pentominoes.
9 Jul 2013
Pentomino P-Tilings: Lower Secondary Mathematics Competition Question
a) For which n ϵ N is it possible to tile a 5 × n rectangle (to cover it without overlap and without gaps)?
b) For which n, m ϵ N is it possible to tile an m × n rectangle (to cover it without overlap and without gaps)?
8 Jul 2013
Pentomino Games and Puzzles: Upper Primary Mathematics
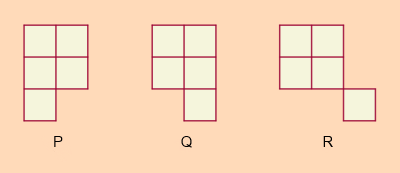
A pentomino is a flat figure made from five unit squares joined along an edge. In the diagram below, figures P and Q are pentominoes but figure R is not.
If you think of each pentomino as a flat shape that can be moved around the plane, then P and Q are two distinct pentominoes. There is no way that we can rotate the P shape so that it matches the Q shape.
How many distinct flat pentominoes can you find?
If you now think of each pentomino as a physical tile that you can hold in your hand, you can easily see that figures P and Q are reflections of each other – you can flip one over and it looks like the other one. These are known as ‘free’ pentominoes.
How many distinct free pentominoes can you make? (As P and Q now count as just one shape, there are fewer free pentominoes than flat ones!)
Warm-up over, let’s get down to the real question! If you are only allowed P and Q pentominoes, in how many ways can you tile the following rectangles, making sure there are no gaps, no overlaps and no overhangs?
a) 5x4 . . . . . . . . . . b) 5x8 . . . . . . . . . . c) 5x10 . . . . . . . . . .
I can hear you thinking:”That’s nice, but where’s the game?!” Here it is: Pentamino (for Windows). Simple to install and... simply frustrating!
If you prefer an online game - and an easier starting level - then try the Pentominoes Game at Scholastic.
Pentominoes also makes an interesting 2 or 3 player game. Taking turns, the aim is to create ‘holes’ in the grid that no pentomino pieces can fit into. However, your opponent is trying to do the same thing. The winner is the last player who has successfully placed a piece on the board.
There are numerous implementations of pentominoes as both a game and a puzzle. If you find one you particularly like, let everyone know in a comment below.
7 Jul 2013
ITYM 2013 Question 6: Upper Secondary Mathematics Competition Question
There are n friends, numbered 1 through n. They are all going to a restaurant for dinner tonight. They are all very good at math, but they are all very strange: the ath friend will be unhappy unless the total cost of the meal is a positive integer, and is divisible by a.
The friends enter the restaurant one at a time. As soon as someone enters the restaurant, if that person is unhappy then the group will call a waiter immediately.
As long as there is at least one unhappy person in the restaurant, one of those unhappy people will buy the lowest-cost item that will make him or her happy. This will continue until nobody in the restaurant is unhappy, and then the waiter will leave. Fortunately, the restaurant sells enough food at every integer price.
The friends could choose to enter the restaurant in any order. After the waiter has been called, if there is more than one unhappy person in the restaurant, any one of those unhappy people could choose to buy something first. The way in which all of those choices are made could have an effect on how many times the group calls a waiter.
For example, if n = 3 and the friends arrive in the order [1, 2, 3], then a waiter will be called three times. (#1 arrives, is unhappy, calls a waiter and buys something costing 1 euro. #2 arrives next, is unhappy, calls a waiter and buys something costing 1 euro. Now nobody is unhappy. #3 arrives next, is unhappy, calls a waiter and buys something costing 1 euro. Now #2 is unhappy and buys something costing 1 euro. Now #3 is unhappy and buys something costing 2 euro, for a total of 6 euros. Finally nobody is unhappy, and a waiter was called three times.) However, if the friends arrive in the order [3, 1, 2], then a waiter will be called 2 times.
1. Denote by Wmax the maximal number of times the friends might call a waiter. Find Wmax or estimate it.
2. Denote by Wmin the minimal number of times the friends might call a waiter. Find Wmin or estimate it.
3. Suppose now that only friends with numbers a1, . . . , ak will come to the restaurant. Find or estimate Wmax and Wmin in this case. Here a1, . . . , ak are distinct positive integers.
4. Denote by W the number of times the friends will call a waiter. Determine the set of all possible values of W . Consider the following cases:
a) The friends are numbered 1 through n.
b) The friends have numbers a1, . . . , ak (distinct positive integers).
5. Denote by U the total number of times the friends were unhappy. Determine the set of all possible values of U in the cases a) and b) above.
6. Suggest and investigate additional directions of research.
This question is from the 2013 ITYM. As of writing, the tournament is ongoing (5-12 July 2013) but a look at the Regulations shows that all the teams have already submitted their solutions (and partial solutions) and that the live tournament is for debating purposes.
If you have participated in any of the International Tournaments of Young Mathematicians then let us know about your experience.
5 Jul 2013
Prize Maths Quiz: Magic X Square (PMQ26)
The image on the left is the magic square depicted in Albrecht Dürer’s famous etching entitled Melencolia I. Using all the whole numbers from 1 to 16, the sum of the numbers in every row, column and diagonal is a constant 34. There are 880 such normal magic 4x4 squares, compared to just the one distinct solution for the 3x3 magic square; it is customary to treat reflections and rotations of an arrangement as just one unique solution. This Dürer magic square is particularly ingenious as the sum of the four corners and the sum of the four central numbers also equal 34. However, today we’re going to look at something slightly different.
Instead of adding the numbers together, we are going to multiply them. Construct, using only distinct positive integers, a 4x4 magic square such that the product of each row, column and diagonal all equal the same number M. Find a square that yields the smallest possible value of M. Include your method of construction.
Have fun!
Hint: the positive integers in the solution cannot be consecutive - try it! - but they do have a deeper kind of order.
4 Jul 2013
JBMO 2013, Problem 4: Middle Secondary Mathematics Competition Question
This is probably my favourite question in this year's JBMO. The hint at the end of the question was printed as part of the question; working through the algebra of the counter-example gives a big clue as to what is going on!
JBMO 2013 Problem 4
Let n be a positive integer. Two players, Alice and Bob, are playing the following game:
= Alice chooses n real numbers, not necessarily distinct.
= Alice writes all pairwise sums on a sheet of paper and gives it to Bob (there are n(n-1)/2 such sums, not necessarily distinct)
= Bob wins if he finds correctly the initial n numbers chosen by Alice with only one guess
Can Bob be sure to win for the following cases?
a) n = 5
b) n = 6
c) n = 8
Justify your answer(s).
[For example, when n = 4, Alice may choose the numbers 1, 5, 7, 9, which have the same pairwise sums as the numbers 2, 4, 6, 10, and hence Bob cannot be sure to win.]
From the Junior Balkan Mathematical Olympiad 2013
[This actually is Problem 4; made an error in the previous post which now has the correct title but a misleading URL.]
JBMO 2013 Problem 1: Middle Secondary Mathematics Competition Question
Find all ordered pairs (a, b) of positive integers for which the numbers
(a3b + 1)/(a + 1) and (b3a + 1)/(b – 1)
are both positive integers.
From the Junior Balkan Mathematical Olympiad 2013
My apologies, but the above question has a typo! The actual question is the following.
Find all ordered pairs (a, b) of positive integers for which the numbers
(a3b – 1)/(a + 1) and (b3a + 1)/(b – 1)
are both positive integers.
Both questions have solutions, and similar methods, but the real question has fewer of them. Feel free to try both. Also, note that 0 is not a positive integer.
(a3b + 1)/(a + 1) and (b3a + 1)/(b – 1)
are both positive integers.
From the Junior Balkan Mathematical Olympiad 2013
My apologies, but the above question has a typo! The actual question is the following.
Find all ordered pairs (a, b) of positive integers for which the numbers
(a3b – 1)/(a + 1) and (b3a + 1)/(b – 1)
are both positive integers.
Both questions have solutions, and similar methods, but the real question has fewer of them. Feel free to try both. Also, note that 0 is not a positive integer.
Nine Points in a Square: Middle Secondary Mathematics Competition Question
Show that given any 9 points inside a square of side 1 we can always find 3 which form a triangle with area less than 1/8.
Junior Balkan Mathematical Olympiad 1997
You may also wish to try different numbers of points within a unit square. Start with just 3 and go all the way up to 9. As in the question above, if you are allowed to pick any 3 points from those given, such that they form a triangle with area less than x, find the minimum value of x in each case.
3 Jul 2013
A Problem With Dates: Middle Secondary Mathematics Competition Question
Imagine that today is Friday 29 March 2013. Daniel’s watch shows the correct day and date. Both the day and date change automatically at midnight. However, if the month has less than 31 days, Daniel must change the date manually at the start of each of those months that follow.
Daniel is a bit absent-minded and forgets to change the date. He notices that he doesn't really need to know the date very often; the day seems to be far more important to him. He therefore decides to do an experiment and never again changes the date on his watch.
Imagine that today is Wednesday 3 July 2013. What day and date are shown on Daniel’s watch?
When will Daniel’s watch show the correct day and date again?
1 Jul 2013
Sharing Coins: Upper Primary Mathematics Competition Question
Jane has 4 coins whose mean value is 4 pence. John has 6 coins whose mean value is 6 pence.
A) If they put all their coins together what is the mean value of their combined total?
B) Also, if their coins can only be of values 1, 2, 5, 10 and 20 pence, is it possible for Jane and John to divide their coins evenly so that they each have the same value? In how many ways can this be done?
A) If they put all their coins together what is the mean value of their combined total?
B) Also, if their coins can only be of values 1, 2, 5, 10 and 20 pence, is it possible for Jane and John to divide their coins evenly so that they each have the same value? In how many ways can this be done?
Subscribe to:
Posts (Atom)







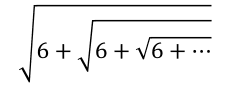


![Pentomino Names [http://commons.wikimedia.org/wiki/File:Pentomino_Naming_Conventions.svg] Pentomino Names [http://commons.wikimedia.org/wiki/File:Pentomino_Naming_Conventions.svg]](http://1.bp.blogspot.com/-RWVqPqe-40s/Ud4T1kAiLgI/AAAAAAAAA5s/g9YrwCRPJT0/s1600/gifted_mathematics_Pentomino_Names.png)